Visual geometric coorelation between nosferatus butterfly and tetryonic deuterium

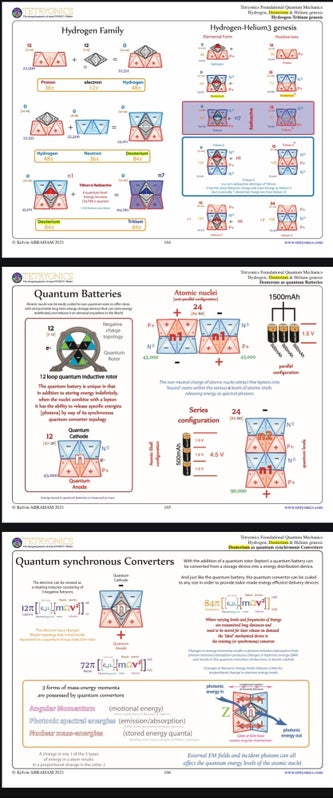
Today I’ve picked up on an interesting visual similarity between the 2D representation of deuterium in this image and Hofstadter's Butterfly. In this instance, time is of the essence and I have not had time to break down the concepts and explore the potential connections.

   The image represents deuterium using a quantum geometric topology, based on the Tetryonic model. It shows a combination of geometric shapes and numerical values representing the composition and properties of deuterium. Hofstadter's Butterfly is a fractal pattern that graphically represents the energy spectrum of electrons in a 2D lattice subjected to a magnetic field. It is a complex, self-similar structure with repeating details. It arises from the mathematical analysis of quantum mechanics in periodic systems. Both the deuterium and Hofstadter's Butterfly involve geometric representations of quantum phenomena. Hofstadter's Butterfly depicts energy levels in a lattice, while the deuterium representation encodes structural information within its geometry. There are likely underlying mathematical structures and symmetries that connect the geometric patterns in both patterns.

  Tetryonic Quantum Geometry, as presented in the image, emphasizes a geometric approach to quantum mechanics. If Tetryonics proposes that nuclear structures have specific geometric forms that relate to their energy states, there could be connections to the mathematical structures that generate Hofstadter's Butterfly. Hofstadter's Butterfly is a fractal, and fractals can appear in various physical systems. Since Tetryonics nuclear models have a fractal nature, this could explain the visual similarity.

Important things to consider when it comes to the visual similarity versus the physical connection is that visual similarity doesn't necessarily imply a direct physical connection. Of course, any connection between the deuterium representation and Hofstadter's Butterfly would require absolute mathematical justification.

The visual similarity between the deuterium representation and Hofstadter's Butterfly is curious. It could potentially point to underlying geometric or mathematical connections between nuclear structures and quantum phenomena. It is still crucial to approach this observation with caution and seek rigorous mathematical and physical explanations.

The images show renderings of Hofstadter's Butterfly, also known as the Gplot, created by Douglas Hofstadter in 1974. The pattern exhibits a high degree of symmetry. The use of colors highlights the complex structure of the fractal.  Further analysis is needed to determine the nature of this color code.

 Again, it's a graphical representation of the energy spectrum of electrons in a two-dimensional square lattice subjected to a perpendicular magnetic field. The fractal nature arises from the interplay between the magnetic field and the lattice periodicity.

  It is a interesting example of how quantum mechanics can lead to intricate mathematical patterns. Since I noticed a visual similarity between this type of pattern and the geometric representation of deuterium in the Tetryonics image I am convinced that science must establish a meaningful connection.

While the visual resemblance between the Hofstadter Butterfly and the Tetryonics depiction of deuterium is striking, claiming it's "undeniably" the same or "statistically impossible" to be a coincidence requires careful consideration and evidence.

Visual similarities can be subjective and influenced by our perception. We must clarify a visual resemblance versus a mathematical identity. To establish a true explanation, we need precise mathematical analysis and comparison of the underlying structures in an independent review.  

Tetryonics uses its own geometric interpretation of nuclear structures, which may not align with standard physics or the mathematical framework that generates Hofstadter's Butterfly. We would need to quantify the visual similarity and calculate the probability of it occurring by chance. We would also need to define what exactly constitutes a "geometric coincidence" in this context.

Without independent evidence or a rigorous mathematical model, the statistical significance of the visual similarity is difficult to assess. Tetryonics' non-standard approach makes it a theory that challenges mainstream physics. This could be why there is no active research on this topic available to the public.

To formulate a meaningful connection, we would need to show that the geometric representation of deuterium in Tetryonics arises from the same or related mathematical equations that generate Hofstadter's Butterfly. We would also need to provide a clear physical interpretation of this connection by overlaying the two images and comparing their peoportions. Potential explanations going beyond coincidence may reveal shared underlying principles or symmetries in quantum mechanics or geometry that give rise to both patterns.

The burden of proof lies on those claiming a direct connection to provide a compelling explanation that goes beyond visual resemblance.